- Derivative Rules. The Derivative tells us the slope of a function at any point. There are rules we can follow to find many derivatives. For example: The slope of a constant value (like 3) is always 0; The slope of a line like 2x is 2, or 3x is 3 etc; and so on. Here are useful rules to help you work out the derivatives of many functions (with.
- 2.6 Derivative Rules: Constant, Sum, Difference,. Constant Multiple. (2.6 includes horizontal tangent lines, equation of the. Normal line, and differentiability of piecewise) 2.7 Derivatives of cos (x), sin (x), e^x, and ln (x) 2.8 The Product Rule. 2.9 The Quotient Rule.
- Unit 3 Derivative Rules Of Compositesap Calculus Integrals
- Unit 3 Derivative Rules Of Compositesap Calculus 2nd Edition
- Unit 3 Derivative Rules Of Compositesap Calculus Solver
PCHS AP CALCULUS. Home Distance Learning Info Assignments & Videos Mr. Zimora's Corner. Unit 3: Applications of Derivatives. Implicit Differentation Part I. Download Packet: AP Calculus AB / IB Math SL Unit 3: Derivative Rules.
The Derivative tells us the slope of a function at any point.
There are rules we can follow to find many derivatives.
For example:
- The slope of a constant value (like 3) is always 0
- The slope of a line like 2x is 2, or 3x is 3 etc
- and so on.
Here are useful rules to help you work out the derivatives of many functions (with examples below). Note: the little mark ’ means 'Derivative of', and f and g are functions.
| Common Functions | Function | Derivative |
|---|---|---|
| Constant | c | 0 |
| Line | x | 1 |
| ax | a | |
| Square | x2 | 2x |
| Square Root | √x | (½)x-½ |
| Exponential | ex | ex |
| ax | ln(a) ax | |
| Logarithms | ln(x) | 1/x |
| loga(x) | 1 / (x ln(a)) | |
| Trigonometry (x is in radians) | sin(x) | cos(x) |
| cos(x) | −sin(x) | |
| tan(x) | sec2(x) | |
| Inverse Trigonometry | sin-1(x) | 1/√(1−x2) |
| cos-1(x) | −1/√(1−x2) | |
| tan-1(x) | 1/(1+x2) | |
| Rules | Function | Derivative |
| Multiplication by constant | cf | cf’ |
| Power Rule | xn | nxn−1 |
| Sum Rule | f + g | f’ + g’ |
| Difference Rule | f - g | f’ − g’ |
| Product Rule | fg | f g’ + f’ g |
| Quotient Rule | f/g | (f’ g − g’ f )/g2 |
| Reciprocal Rule | 1/f | −f’/f2 |
| Chain Rule (as 'Composition of Functions') | f º g | (f’ º g) × g’ |
| Chain Rule (using ’ ) | f(g(x)) | f’(g(x))g’(x) |
| Chain Rule (using ddx ) | dydx = dydududx | |

'The derivative of' is also written ddx
So ddxsin(x) and sin(x)’ both mean 'The derivative of sin(x)'
Examples
Example: what is the derivative of sin(x) ?
From the table above it is listed as being cos(x)
It can be written as:
sin(x) = cos(x)
Or:
sin(x)’ = cos(x)
Power Rule
Example: What is x3 ?
The question is asking 'what is the derivative of x3 ?'
We can use the Power Rule, where n=3:
xn = nxn−1
x3 = 3x3−1 = 3x2
(In other words the derivative of x3 is 3x2)
So it is simply this:
3x^2'>
'multiply by power
then reduce power by 1'
It can also be used in cases like this:
Example: What is (1/x) ?
1/x is also x-1
We can use the Power Rule, where n = −1:
xn = nxn−1
x−1 = −1x−1−1
= −x−2
= −1x2
So we just did this:
-x^-2'>
which simplifies to −1/x2
Multiplication by constant
Example: What is 5x3 ?
the derivative of cf = cf’
the derivative of 5f = 5f’
We know (from the Power Rule):
x3 = 3x3−1 = 3x2
So:
5x3 = 5x3 = 5 × 3x2 = 15x2
Sum Rule
Example: What is the derivative of x2+x3 ?
The Sum Rule says:
the derivative of f + g = f’ + g’

So we can work out each derivative separately and then add them.
Using the Power Rule:
- x2 = 2x
- x3 = 3x2
And so:
the derivative of x2 + x3 = 2x + 3x2
Difference Rule
It doesn't have to be x, we can differentiate with respect to, for example, v:
Example: What is (v3−v4) ?
The Difference Rule says
the derivative of f − g = f’ − g’
So we can work out each derivative separately and then subtract them.
Using the Power Rule:
- v3 = 3v2
- v4 = 4v3

And so:
the derivative of v3 − v4 = 3v2 − 4v3
Sum, Difference, Constant Multiplication And Power Rules
Example: What is (5z2 + z3 − 7z4) ?
Using the Power Rule:
- z2 = 2z
- z3 = 3z2
- z4 = 4z3
And so:
(5z2 + z3 − 7z4) = 5 × 2z + 3z2 − 7 × 4z3 = 10z + 3z2 − 28z3
Product Rule
Example: What is the derivative of cos(x)sin(x) ?
The Product Rule says:
the derivative of fg = f g’ + f’ g
In our case:
- f = cos
- g = sin
We know (from the table above):
- cos(x) = −sin(x)
- sin(x) = cos(x)
So:
the derivative of cos(x)sin(x) = cos(x)cos(x) − sin(x)sin(x)
= cos2(x) − sin2(x)
Quotient Rule
To help you remember:
(fg)’ = gf’ − fg’g2
The derivative of 'High over Low' is:
'Low dHigh minus High dLow, over the line and square the Low'
Example: What is the derivative of cos(x)/x ?
In our case:
- f = cos
- g = x
We know (from the table above):
- f' = −sin(x)
- g' = 1
So:
the derivative of cos(x)x = Low dHigh minus High dLowover the line and square the Low
= x(−sin(x)) − cos(x)(1)x2
= −xsin(x) + cos(x)x2
Reciprocal Rule
Example: What is (1/x) ?
The Reciprocal Rule says:
the derivative of 1f = −f’f2
With f(x)= x, we know that f’(x) = 1
Unit 3 Derivative Rules Of Compositesap Calculus Integrals
So:
the derivative of 1x = −1x2
Which is the same result we got above using the Power Rule.
Chain Rule
Example: What is ddxsin(x2) ?
sin(x2) is made up of sin() and x2:
- f(g) = sin(g)
- g(x) = x2
The Chain Rule says:
the derivative of f(g(x)) = f'(g(x))g'(x)
The individual derivatives are:
- f'(g) = cos(g)
- g'(x) = 2x
So:
ddxsin(x2) = cos(g(x)) (2x)
= 2x cos(x2)
Another way of writing the Chain Rule is: dydx = dydududx
Let's do the previous example again using that formula:
Example: What is ddxsin(x2) ?
dydx = dydududx
Have u = x2, so y = sin(u):
ddx sin(x2) = ddusin(u)ddxx2
Differentiate each:
ddx sin(x2) = cos(u) (2x)
Substitue back u = x2 and simplify:
ddx sin(x2) = 2x cos(x2)
Same result as before (thank goodness!)
Another couple of examples of the Chain Rule:
Example: What is (1/cos(x)) ?
1/cos(x) is made up of 1/g and cos():
- f(g) = 1/g
- g(x) = cos(x)
The Chain Rule says:
the derivative of f(g(x)) = f’(g(x))g’(x)
The individual derivatives are:
- f'(g) = −1/(g2)
- g'(x) = −sin(x)
So:
(1/cos(x))’ = −1/(g(x))2 × −sin(x)
= sin(x)/cos2(x)
Note: sin(x)/cos2(x) is also tan(x)/cos(x), or many other forms.
Example: What is (5x−2)3 ?
The Chain Rule says:
the derivative of f(g(x)) = f’(g(x))g’(x)
(5x-2)3 is made up of g3 and 5x-2:
- f(g) = g3
- g(x) = 5x−2
The individual derivatives are:
- f'(g) = 3g2 (by the Power Rule)
- g'(x) = 5
So:
(5x−2)3 = 3g(x)2 × 5 = 15(5x−2)2
You know you’ll have to know your derivatives inside and out in order to score high on the AP Calculus exam. But what are the most common derivatives you’ll see on the test? In this short article, we’ll let you in on the secret!
Know the Basics
First of all, it’s very important to have the basics down. That includes: the Power Rule, Product Rule, Quotient Rule, and Chain Rule, among others.
Don’t forget about those special functions either. You’ll have to memorize the derivative rules for trig, exponential, and logarithmic functions.
Derivatives of trigonometric, exponential, and logarithmic functions
Less common, but no less important are the rules for inverse trig functions.
Check out Calculus Review: Derivative Rules and Derivatives on the AP Calculus AB & BC Exams: A Refresher for more.
Common Derivatives
Now let’s take a look at a few problems involving common derivatives that are modeled after actual AP Calculus problems.
Problem 1
Unit 3 Derivative Rules Of Compositesap Calculus 2nd Edition
Find The tangent line to the curve f(x) = x4 + 3x – 10 at the point (1, -6).
Solution
To find a tangent line, first take the derivative. Here, we have to use the Power Rule and Sum/Difference Rule.
f '(x) = 4x3 + 3
Then you can find the slope and the equation of the tangent line.
Slope at x = 1: f '(1) = 4(1)3 + 3 = 7
y – (-6) = 7(x – 1)
y = 7x – 13.
Problem 2
The position of a particle moving along the x-axis at time t is x(t) = sin( cos( 4t ) ), for 0 ≤ t ≤ π. Find
the velocity at time t = π/8.
Solution
Find velocity by taking the derivative of the position function. Be careful — we need two applications of the Chain Rule for this one!
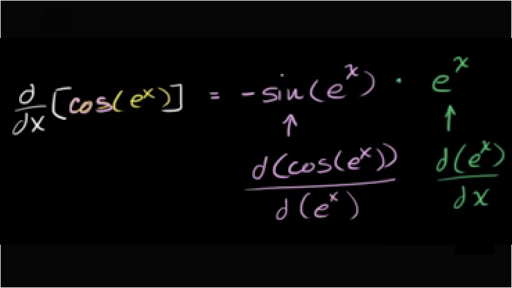
v(t) = x '(t) = cos( cos( 4t ) ) ( -sin( 4t ) ) (4)
At time t = π/8, the velocity is equal to:
v(π/8) = cos( cos( 4(π/8) ) ) ( -sin( 4(π/8) ) ) (4) = cos( cos( π/2 ) ) ( -sin( π/2 ) ) (4)
= cos(0)(-1)(4) = (1)(-1)(4) = -4.
Problem 3
Find all inflection points of the curve defined by .
Solution
You can find inflection points by taking the second derivative. Of course, you must take the first derivative first. Don’t forget to rewrite your radical as a power and use Chain Rule.
Use Quotient Rule to help find the second derivative.
After simplification, we find that the second derivative is never equal to 0 and never undefined. Therefore this function has no inflection points.
Problem 4
Unit 3 Derivative Rules Of Compositesap Calculus Solver
Solution
You’ll need both the Product and Chain Rules for this one.
Problem 5
Suppose g(x) = e4x + 5. At what value of x is the slope of the tangent line to g(x) equal to 3?
Solution
Again, the slope of the tangent line is equal to a derivative value.
g '(x) = 4e4x
Set g '(x) = 3 and solve:
4e4x = 3
e4x = 3/4
4x = ln(3/4)
x = ln(3/4) / 4 ≈ -0.0719
Summary
The five problems above represent just a small sampling of what you’ll find on an AP Calculus AB or BC exam. Nevertheless, the majority of problems involving derivatives do tend to fall into these basic formats.
- Know your basic rules, especially the Chain Rule.
- Memorize the derivatives of the special functions.
- And above all, know which tools apply in each situation.
Good luck on the exam!
Improve your SAT or ACT score, guaranteed. Start your 1 Week Free Trial of Magoosh SAT Prep or your 1 Week Free Trial of Magoosh ACT Prep today!
More from Magoosh

About Shaun Ault
Shaun earned his Ph. D. in mathematics from The Ohio State University in 2008 (Go Bucks!!). He received his BA in Mathematics with a minor in computer science from Oberlin College in 2002. In addition, Shaun earned a B. Mus. from the Oberlin Conservatory in the same year, with a major in music composition. Shaun still loves music -- almost as much as math! -- and he (thinks he) can play piano, guitar, and bass. Shaun has taught and tutored students in mathematics for about a decade, and hopes his experience can help you to succeed!
Leave a Reply
Magoosh blog comment policy: To create the best experience for our readers, we will approve and respond to comments that are relevant to the article, general enough to be helpful to other students, concise, and well-written! :) If your comment was not approved, it likely did not adhere to these guidelines. If you are a Premium Magoosh student and would like more personalized service, you can use the Help tab on the Magoosh dashboard. Thanks!
